In a purely resistive AC circuit, voltage and current waveforms are in step (or in phase), changing polarity at the same instant in each cycle. All the power entering the loads is consumed. Where reactive loads are present, such as withcapacitors or inductors, energy storage in the loads result in a time difference between the current and voltage waveforms. During each cycle of the AC voltage, extra energy, in addition to any energy consumed in the load, is temporarily stored in the load in electric or magnetic fields, and then returned to the power grid a fraction of a second later in the cycle. The "ebb and flow" of this nonproductive power increases the current in the line. Thus, a circuit with a low power factor will use higher currents to transfer a given quantity of real power than a circuit with a high power factor. A linear load does not change the shape of the waveform of the current, but may change the relative timing (phase) between voltage and current.
Circuits containing purely resistive heating elements (filament lamps, strip heaters, cooking stoves, etc.) have a power factor of 1.0. Circuits containing inductive or capacitive elements (electric motors, solenoid valves, lamp ballasts, and others ) often have a power factor below 1.0.
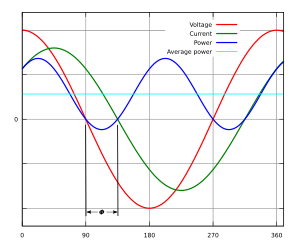
Instantaneous and average power calculated from AC voltage and current with a unity power factor (φ=0, cosφ=1). Since the blue line is above the axis, all power is real power consumed by the load.
Definition and calculation:
AC power flow has the three components: real power (also known as active power) (P), measured in watts (W); apparent power (S), measured in volt-amperes (VA); and reactive power (Q), measured in reactive volt-amperes(var).[3]
The power factor is defined as:
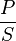 .
.
In the case of a perfectly sinusoidal waveform, P, Q and S can be expressed as vectors that form a vector triangle such that:
If 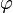 is the phase angle between the current and voltage, then the power factor is equal to the cosine of the angle,
is the phase angle between the current and voltage, then the power factor is equal to the cosine of the angle, 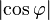 , and:
, and:
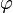 is the phase angle between the current and voltage, then the power factor is equal to the cosine of the angle,
is the phase angle between the current and voltage, then the power factor is equal to the cosine of the angle, 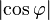 , and:
, and:Since the units are consistent, the power factor is by definition a dimensionless number between 0 and 1. When power factor is equal to 0, the energy flow is entirely reactive, and stored energy in the load returns to the source on each cycle. When the power factor is 1, all the energy supplied by the source is consumed by the load. Power factors are usually stated as "leading" or "lagging" to show the sign of the phase angle.
If a purely resistive load is connected to a power supply, current and voltage will change polarity in step, the power factor will be unity (1), and the electrical energy flows in a single direction across the network in each cycle. Inductive loads such as transformers and motors (any type of wound coil) consume reactive power with current waveform lagging the voltage. Capacitive loads such as capacitor banks or buried cable generate reactive power with current phase leading the voltage. Both types of loads will absorb energy during part of the AC cycle, which is stored in the device's magnetic or electric field, only to return this energy back to the source during the rest of the cycle.
For example, to get 1 kW of real power, if the power factor is unity, 1 kVA of apparent power needs to be transferred (1 kW ÷ 1 = 1 kVA). At low values of power factor, more apparent power needs to be transferred to get the same real power. To get 1 kW of real power at 0.2 power factor, 5 kVA of apparent power needs to be transferred (1 kW ÷ 0.2 = 5 kVA). This apparent power must be produced and transmitted to the load in the conventional fashion, and is subject to the usual distributed losses in the production and transmission processes.
Electrical loads consuming alternating current power consume both real power and reactive power. The vector sum of real and reactive power is the apparent power. The presence of reactive power causes the real power to be less than the apparent power, and so, the electric load has a power factor of less than 1.
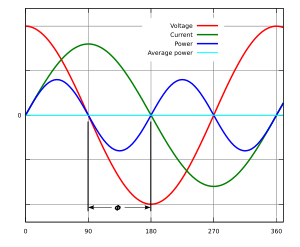
Instantaneous and average power calculated from AC voltage and current with a zero power factor (φ=90, cosφ=0). The blue line shows all the power is stored temporarily in the load during the first quarter cycle and returned to the grid during the second quarter cycle, so no real power is consumed.


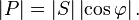
No comments:
Post a Comment